- CFA Exams
- 2025 Level II
- Topic 6. Fixed Income
- Learning Module 27. The Arbitrage-Free Valuation Framework
- Subject 1. Arbitrage-Free Valuation for an Option-Free Bond
Why should I choose AnalystNotes?
AnalystNotes specializes in helping candidates pass. Period.
Subject 1. Arbitrage-Free Valuation for an Option-Free Bond PDF Download
The traditional valuation approach uses a single interest rate to discount all of a bond's cash flows. It views all cash flows of a bond as the same, regardless of the timing of those cash flows. In reality, however, each individual cash flow of the bond is unique. Therefore, using a single discount rate in the bond valuation model may result in a mis-priced bond, thereby creating arbitrage opportunities.V0 = C/(1 + i1) + C/(1 + i2)2 + C/(1 + i3)3 + C/(1 + i4)4 + ... + (C + P)/(1 + iN)N 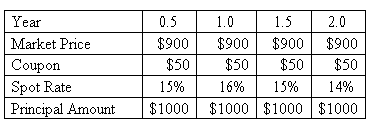
The arbitrage-free approach values a bond as a package of cash flows, with each cash flow viewed as a zero-coupon bond and each cash flow discounted at its own unique discount rate.
- The theoretical Treasury zero-coupon rates are called Treasury spot rates.
- These rates are used to discount the cash flows to get the arbitrage-free value of a bond.
The arbitrage-free approach has three steps:
- Take each individual cash flow of a coupon as a stand-alone zero-coupon bond. Each cash flow is the face value of the corresponding zero.
- Value each zero-coupon bond by discounting its cash flow at the corresponding spot rate.
- Add up the value of each zero to calculate the total value of the zero-coupon bond portfolio.
The process of stripping and reconstitution forces the price of a bond toward its arbitrage-free value so that no arbitrage profit is possible.
Using a zero-coupon bond, an investor can create a synthetic coupon bond that has the same cash flows as the bond being valued. For example, an investor can create a synthetic 2-year T-bond by buying 4 zeros, three with a face value of $50 and one with a face value of $1,050. The synthetic bond is valued by discounting each zero at the appropriate spot rate. This is exactly how the arbitrage-free approach values a coupon bond. Thus, the synthetic bond and the corresponding coupon bond should have the same value. If they don't, there is an arbitrage opportunity available. The investor can buy the one with the lower price and sell the one with the higher price, making a riskless profit.
Example
Michael Merley, a dealer for Smith, Barney, Rubble investment house, has observed a 2-year, 10% coupon Treasury security selling for $900. Michael knows that the annualized spot rates for Treasury securities range from 14 - 16% (shown below).

How can Michael take advantage of this situation?
Answer: He should buy the 2 year Treasury and strip and sell the coupons and principal at the spot rates.
The solution can be achieved in several ways.
One approach is to calculate the implied YTM on the 2-year coupon security. The YTM can be calculated by: n = 4pv = -900fv = 1000pmt = 50. Solve for i = 8.04 * 2 (for semi-annual interest) = 16.04%. Since the YTM is higher than any individual spot rate, the 2-year Treasury is priced low relative to the spot rates (i.e., higher yields). Thus, Michael would want to buy the 2-year Treasury and sell at the lower yields (i.e., higher price). This can be accomplished by stripping the 2-year coupon and principal payments and selling at the spot rates.
For proof of the arbitrage opportunity, Michael may discount each annual cash flow at the prevailing spot rate. This provides a discounted cash flow stream of $930.67. Given the availability of the bond selling in the marketplace at $900, an arbitrageur can purchase the bond at $900, strip the coupons and principal for $930.67 and earn a risk-free profit of $30.67. In reality, such a large risk-free profit is unlikely given the number of market participants, however this provides the theoretical foundations for how an arbitrage profit situation operates. Discounted Cash flows: Period 1 = 46.51; Period 2 = 42.87; Period 3 = 40.25; Period 4 = 801.04; Total = $930.67.
User Contributed Comments 7
| User | Comment |
|---|---|
| myron | The traditional approach discounts each cash flow at the same rate. The arbitrage-free value is the value of a bond based on spot rates applied to each corresponding cash flow. It computes the value of a security assuming that it is converted into a series of zero-coupon bonds. |
| connor15 | A fundamental principal of valuation is that the value of any financial asset is equal to the present value of its expected future cash flows. |
| cfa2017 | The replicating portfolio of a coupon bond, in turn, is the portfolio of zero discount bonds. Thus, one of the important features of the calibration model is that it yields prices on option-free bonds that are arbitrage free. |
| ashish100 | Can someone explain the discounting part so that the next person who is confused can get it right? fml |
| noname | I tried the below and got similar but not exact answers for the last example Discounted Cf = cf/(1+spot rate)^t |
| darbyland | After stripping bond into 4 different cash flows, I got 938-ish |
| davidt87 | Ashish you're making me feel way better about this section. Calculation is right: Coupon at t=0.5: $50/(1+(0.15/2))^1 = $46.511 Coupon at t=1.0: $50/(1+(0.16/2))^2 = $42.866 Coupon at t=1.5: $50/(1+(0.15/2))^3 = $40.248 Coupon at t=2.0: $1050/(1+(0.14/2))^4 = $801.039 Stripped Value = $930.665 |

I used your notes and passed ... highly recommended!

Lauren
My Own Flashcard
No flashcard found. Add a private flashcard for the subject.
Add