- CFA Exams
- 2025 Level I
- Topic 1. Quantitative Methods
- Learning Module 8. Hypothesis Testing
- Subject 11. Tests Concerning the Equality of Two Variances (F-Test)
Why should I choose AnalystNotes?
AnalystNotes specializes in helping candidates pass. Period.
Subject 11. Tests Concerning the Equality of Two Variances (F-Test) PDF Download
Just as in the case of means of populations, now it's time to look at the test for equality of variances of two normally distributed populations. Label the populations 1 and 2, as has always been done. The choice of labeling is arbitrary.
The test statistic is
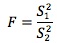 , where (s1)2 and (s2)2 are the sample variances from two samples, which have n1 and n2 observations in each. The samples are random, independent of each other, and generated by normally distributed populations.
, where (s1)2 and (s2)2 are the sample variances from two samples, which have n1 and n2 observations in each. The samples are random, independent of each other, and generated by normally distributed populations.
The F stands for Fisher, the name of the person who formulated this distribution.
This test statistic will have n1 - 1 degrees of freedom in the numerator and n2 - 1 degrees of freedom in the denominator.
The values n1 - 1 and n2 - 1 are also the divisors used in calculating (s1)2 and (s2)2, respectively.
Another point of interest is that, under H0, the ratio σ12/σ22 is 1.
A F-test checks whether or not the ratio of the test statistic, (s1)2/(s2)2, is close to 1. If it is, you will obtain a non-rejection of H0; if not, you will obtain a rejection of H0.
For this reason, it is common practice to use the larger of the two ratios (s1)2/(s2)2 or (s2)2/(s1)2, as the actual test statistic. So, whichever of (s1)2 and (s2)2 is larger should go in the numerator, with the smaller number in the denominator.
If these values are equal, it makes no difference which value goes on top, as the test statistic will be 1 in either case.
Like the chi-square distribution:
- the F-distribution is bounded below by zero, and therefore values of the F-distribution test statistic cannot be negative.
- the F-distribution is asymmetrical.
- the F-distribution is a family of distributions.
- the F-test is not very robust when assumptions are violated.
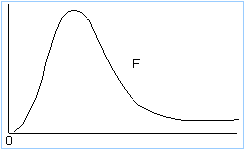
Unlike the chi-square distribution, the F-distribution is determined by two parameters: the degrees of freedom in the numerator and the degrees of freedom in the denominator. Note that F(8,6) refers to an F-distribution with 8 numerator and 7 denominator degrees of freedom.
Another area in which F-tests differ from chi-square tests is that, for F-tests, the rejection region is always in the right tail of the graph, never the left tail.
This presents few difficulties. It does mean that if you are conducting a one-sided test, the full value goes into the right tail, whereas if you are conducting a two-sided test, you divide by 2 to obtain the area in the right tail. The remaining half of the area effectively falls away because of the convention of putting the larger sample variance on top in the test statistic. Provided you do this, you will have no problems here.
Critical values for this distribution are found in F-tables. In order to find the required value, you should look up the value that corresponds with the correct numerator and denominator degrees of freedom.
Example
You are an investor skeptical of the value added by Jupiter Fund, an actively managed large-cap mutual fund. An examination of the prospectus reveals that it has tracked its target index fund very closely over the past 10 years, yet it incurs a 1.51% annual expense fee, far in excess of what one might pay to invest in a large-cap index fund. You wonder if Jupiter achieves a smaller standard deviation in asset values to justify its higher costs.
You obtain monthly net asset values for both Jupiter Fund and the market index for the past 10 years. You find that Jupiter fund has a sample variance of 0.0016 and that the market index has a sample variance of 0.0025. At the alpha = 0.05 significance value, is the variance for Jupiter statistically less than that for the market index fund? Assume independence of observations and normally distributed populations.
Solution
Your null hypothesis is:
H0: (σ1)2 ≤ (σ2)2 versus H1: (σ1)2 > (σ2)2 where Jupiter Fund is population 2. This may seem counter-intuitive; the explanation follows below.
The test is a one-tailed F-test, with 119 degrees of freedom in both the numerator and the denominator. Using the convention of placing the higher variance term in the numerator, you get an F-value of 0.0025/0.0016 = 25/16 = 1.5625.
Note that by putting the higher variance term in the numerator, you are, in effect, saying that the market index is population 1. If the statistic is large, then you are finding evidence for the alternative hypothesis, that (σ1)2 > (σ2)2.
Your critical value will be found with the area in the right tail equal to 0.05. The table in Appendix D contains entries for 60 and 120 degrees of freedom; you can interpolate, but the difference is so small that you can just use the values at 120 degrees of freedom. The critical value is thus 1.35, and your test statistic exceeds this. Thus, you will reject the null hypothesis and conclude that the market index does have significantly larger variance (or alternatively, that Jupiter Fund has significantly smaller variance).
User Contributed Comments 9
| User | Comment |
|---|---|
| vincenthuang | F test is always greater than or equel to 1 |
| soniamoren | The higher varieance value is always in the numerator |
| epizi | where is the degree of freedoms in the question? |
| thanks | The degree of freedom is the total number of months minus 1 (i.e 10 yrs * 12 = 120 mths -1 = 119). The degree of freedom is then used to obtain the critical value. |
| ddrmax | This is a one-tailed test since we are testing less than. |
| Shaan23 | Notes: F(8,6) means 8 numerator and 7 denominator DF. that doesnt seem right. |
| Shaan23 | Read my note above. Thats wrong. It's 8 numerator and 6 denominator DF. |
| rjdelong | yes it is a one-tail test which is why they didn't divide the .05 in half, we want to know if it is less than the index. |
| Allen88 | The degrees of freedom are 119 for both the numerator and denominator, therefore the critical value should be 1.26 F(119,119), rather than 1.35 at F(119, 60). |

I passed! I did not get a chance to tell you before the exam - but your site was excellent. I will definitely take it next year for Level II.

Tamara Schultz
My Own Flashcard
No flashcard found. Add a private flashcard for the subject.
Add