Swaps are derivative securities in the form of agreements between two counterparties to exchange cash flows over a period of time, depending on the values of specified market variables.

Example

Party A agrees to pay a fixed rate of interest on $10 million each year for 3 years to Party B. In return, Party B agrees to pay a floating rate of interest on $10 million each year for 3 years to Party A.

A swap involves a series of payments over its tenor, and can be considered a series of forward contracts. In contrast, forwards, futures and options only involve a single payment or two payments (i.e., when the option is purchased and when it is exercised).

In general, neither party pays any money to the other at the initiation of a swap. A swap has zero value at the start.

A swap can be viewed as combining a series of forward contracts into a single transaction. However, there are some small differences. For example, swaps are a series of equal fixed payments, whereas the component contracts of a series of forward contracts would almost always be priced at different fixed rates. In this context we often refer to a swap as a series of off-market forward contracts, reflecting the fact that the implicit forward contracts that make up the swap are all priced at the swap fixed rate and not at the rate at which they would normally be priced in the market.

Another similarity is that both forward contracts and swap contracts are traded over the counter.

To understand swaps, let us look at an example.

On December 15 XYZ enters into a $50 million NP swap with ABSwaps. Payments will be on the 15th of March, June, September, and December for one year, based on LIBOR. XYZ will pay 7.5% fixed and ABSwaps will pay LIBOR. Interest is based on exact day counts and 360 days (30 per month).

In general, the cash flow to the fixed payer will be:

Notional principal x (LIBOR - Fixed rate) x (Days / 360 or 365)

• The payments in this swap are: 50,000,000 x (LIBOR - 0.075) x (days/360). Note that payments are netted.
• We denote L_i(m) as the m-day LIBOR on day i. If i = 1, we are referring to 90 days after day 0 in this example, and L₁(90) is the 90-day LIBOR on day 90.
• q represents the factor (days/360).

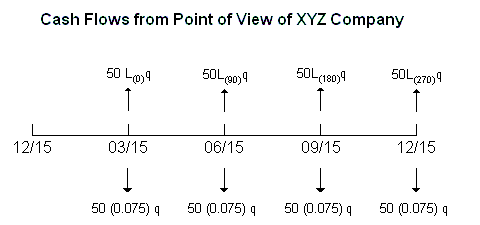
After-the-fact payments:

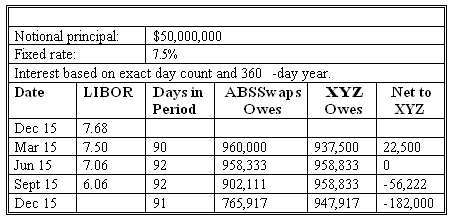
Note: This combination of LIBORS on the above dates represents only one of an infinite number of possible outcomes to the swap. They are used to illustrate how the payments are determined and not the likely results.

Pricing an Interest Rate Swap

Remember that swap pricing means determining the fixed rate at the start of the swap.

We denote PV_0,ti(1) as the present value factor on a zero-coupon instrument paying $1 at its maturity date. The price of a LIBOR zero coupon bond with a maturity of t_i days is 1/{1 + L₀(t_i) * (t_i/360)}. As an example, to discount payments 180 days later, we multiply the payment amount by the following factor: PV_{0, 180}(1) = 1 / {1 + [L₀(180) x (180/360)]}. We can think of this discount factor as the value of a spot LIBOR deposit that pays $1 after 180 days.

On day 0, the floating rate is set for the first period and the interest is to be paid at that rate on day 90. Then, on day 90, the rate is set for the second period and the interest is paid on day 180. This process continues so that on day 270 the rate is set for the last period, and the final interest payment and the principal are paid on day 360. Let the principal be 1.0.

Starting at the maturity date and working back, we can see that the value is par at the start and on each coupon reset date.

• Suppose today is day 270, and the LIBOR today is L₂₇₀(90). Remember that this rate is the 90-day LIBOR in the market at the time. Looking ahead to day 360, we anticipate receiving 1.0, the final principal payment, plus L₂₇₀(90) x 90/360. What is the value of this amount on day 270? We would discount it by the appropriate 90-day LIBOR in the following manner:
  Value on day 270 = (Payment on day 360) x (One-period discount factor) = [1.0 + L₂₇₀(90) x (90/360)] x {1 / [1 + (L₂₇₀(90) x (90/360))]} = 1.0

• We continue this procedure, stepping back until we reach time 0.

Payments in an interest rate swap do not include an initial or final exchange of notional principals. If we introduce the notional principal payments as though they were actually made, we have not done any harm. The cash flows on the swap are still the same. However, by adding the notional principals, we can separate the cash flow streams of an interest rate swap into those of a fixed-rate bond and a floating-rate bond.

The value of a swap is: V = value of fixed bond (FB) - Value of floating bond (VB).

• The value of floating bond: VB = 1 (at time 0 or a payment date).
• The value of fixed bond is simply the sum of the present value factors for each payment.

To price the swap at the start, set V to zero.

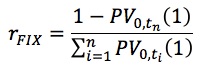
The expression PV_{0, tn}(1) is the present value factor for the final hypothetical notional principal payment of 1.

In the above example, suppose the term structure of LIBOR at the start of the contract is:

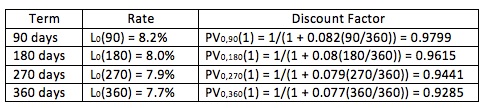
The annualized fixed rate would, therefore, be R = (360/90) [(1 - 0.9285) / (0.9799 + 0.9615 + 0.9441 + 0.9285)] = 0.075.

Thus, the rate would be 7.5%.

Valuing an Interest Rate Swap

The value of a fixed rate swap at some future point in time, t, is simply the sum of the present value of the difference in fixed swap rates multiplied by the stated notional amount (denoted NA), or:

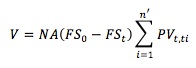
where:

• FS₀ is the fixed rate established at the start of the swap.
• FS_t is the fixed rate established at Time t.